The Basic Physics and Mathematics of Table Tennis

Thanks to Jonathan Roberts for contributing this article.

Firstly, a very brief introduction to the mathematics that is used to describe Table Tennis. There are a handful of formulae that are used, which a man called Sir Isaac Newton derived in his monumental work Philosophae Naturalis Principia Mathematica. Incidentally, this work is generally regarded as the single most important work ever written in the history of science, and I regard Newton as the greatest scientist to have ever lived.

It accurately explains how objects move from the scale of interstellar objects (galaxies, stars, planets, SERIOUSLY BIG STUFF etc.), down to things on the scale of about 1000th of a millimetre or 1 micron. After that, this model of the universe starts to break down and you need to go to Quantum Theory and Relativity, which involves FRIGHTENING Mathematics and Physics to use.

Anyway, this is the Physics and Mathematics of Table Tennis in the Newtonian Universe.

The basic formulae to be used here are:
P = W÷t
W = Fs
F = ma
a = (v – u)÷t note: This is usually rearranged to v = u + at
T = rF

Note: When two letters are next to each other it means multiplication. This is the correct notation. Take the second formula as an example, W = Fs
This is expressed as W = F multiplied by s or W = F x s.

Where:

P=	Power (The amount of oomph that is applied)
W=	Work (The amount of energy that is consumed)
t=	Time (Length of time the Power is applied for)
F=	Force (Basically the amount of grunt the shot has. Similar to P but subtly different)
s=	Displacement (this essentially translates to Distance, except under certain circumstances)
m=	Mass (weight of the ball, fixed at 2.7g)
a=	Acceleration (change in velocity over a given time period)
v=	Velocity (speed of the shot)
u=	Initial Velocity (how fast the ball is hit at you)
T=	Torque (The amount of Turning Force that is applied)
r=	Radius (the length from the middle of a circle, to the perimeter.)

P = W÷t
In order to gain more power in your shots, you have to do more work or take less time in your shots. The time in a shot refers to the time the ball is in contact with the racquet which is fixed at approximately 0.003 seconds. Therefore, in order to increase the Work done, the second equation must be examined:

W = Fs
If the amount of Force is increased, then the Work coefficient is increased. The other way is to increase the Displacement, but that cannot be done as the length of the Table is fixed (technically, lobbing or looping the ball will increase the Work done, as the ball has to cover a larger distance than ball that barely clears the net). In order to increase Force, the third equation must be examined.

F = ma
In order to increase the Force, the Mass of the ball needs to be increased which is impossible, or the Acceleration needs to be increased. In order to increase the acceleration, we analyse the fifth equation.

a = (v – u)÷t
The result of the calculation between the brackets must be calculated first (it’s a mathematical law). Therefore you want to maximise the acceleration, minimise the initial velocity. In order to maximise the velocity, you have to hit the ball as hard as you can. The initial velocity is something you have no control over, as it is how hard the opposition hits the ball at you. However, as the initial velocity is coming towards you, its value is negative. So it is actually added to your velocity, as subtracting a negative number actually means you add the two terms (another mathematical law). The time remains fixed, for the reason explained above.

Therefore this demonstrates why the harder you hit the ball, the more Power it will have.

But, speed is not everything in Table Tennis. There is spin, which will now be discussed.

All about spin

A ball that is spinning is always easier to return than a ball that is not spinning because a ball that spins has stability at range. The frontiersmen of America had worked this out and used it with their rifles. If you look down the barrel of a rifle, you’ll see it has what are called ‘lands’ down the barrel. These are grooves cut into the barrel that twist in one direction, causing the bullet to spin. This gives the projectile stability at range. Without the lands, the projectile would stray off course after about 50 metres and certainly by a hundred. For history buffs, rifling was discovered and exploited during the American War of Independence.

To understand spin, an understanding of what’s known as air speed and relative air speed is required.

Air speed: This is simply the speed at which an object moves through the air. A top pennants player can smash the ball at about 200 kilometres per hour. This is the speed of the ball relative to a stationary object (the table, the umpire’s chair …, as long is it isn’t moving, or else you start to get into the beginnings of Einstein’s Theory of Relativity, which I’m NOT going into here). If the air itself is moving, then relative air speed is used.

Relative Air Speed: This takes into account any wind that the ball is travelling through. If for instance, you were to smash the ball (with an air speed of 200 km/hr) into headwinds of 10 km/hr, then the relative air speed would be 210 km/hr. If on the other hand you had the wind blowing behind you at 10 km/hr, the relative air speed would be 190 km/hr.

When wind occurs at an angle you introduce what’s known as a vector term. This means the angle of the wind only partially affects the ball.

The mathematics is as follows:

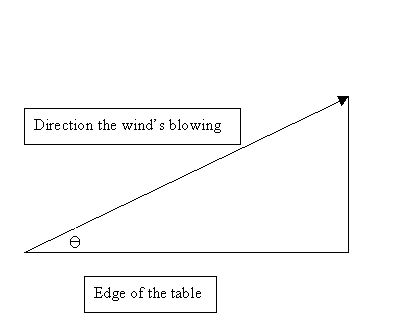
The above triangle shows a vector diagram of the direction (the angle, Ø, or Theta) and velocity (the length of the line) the wind is blowing. Through this diagram, a number can be derived to represent the wind speed on the ball.

Sine Ø =	Short line ÷ Direction the wind’s blowing
Direction and magnitude of wind =	Short line ÷ Sine Ø

This isn’t really an important factor in table tennis, as wind speed is usually negligible, due playing indoors, unless you have a fan on in the same room.

To fully understand the concept of spinning the ball, a look at what happens when topspin, underspin and sidespin is applied to the ball must be analysed.

A heavily stylised topspun ball:

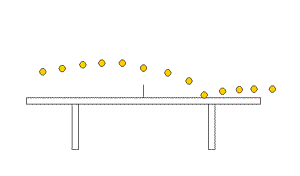
The ball will tend to come off the table flatter and faster than if it was just blocked back. The ball also has a tendency to drop suddenly, Think of the effect a high loop has on the ball. This is an extreme example of topspin in use.

A heavily stylised underspun ball:

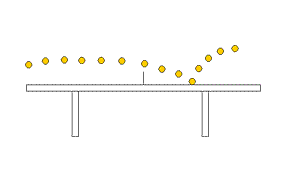
The ball will tend to float on to the other side of the table. It has a tendency to stay high for longer. When it bounces, the ball tends to kick up off the table. A late chop taken far from the table that just clears the net will demonstrate this.

A heavily stylised sidespun ball:

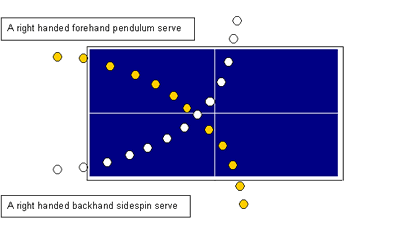
With sidespin, the ball will tend to curl either left or right. This is clearly demonstrated in service. A forehand pendulum serve will tend to curl away to the opposition’s left, whereas a backhand sidespin serve will tend to curl away to the opposition’s right (assuming you’re a right hander).

Why does spin behave the way it does?

To fully understand the dynamics of spin, the relative air speed in relation to the ball’s speed must be examined. If you spin the ball (in the diagram below it is top spun), then at a certain point, it will have a minimum relative air speed. At the point where there is a minimum relative air speed, a slight vacuum occurs.

A topspun ball moving through air:

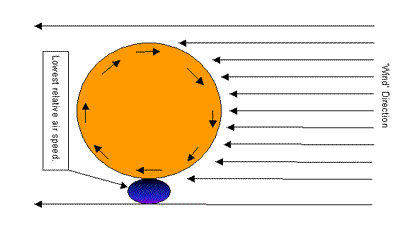
The wind is in quotes, because it is created by the direction the ball is travelling. It’s the same as riding a bike on a still day. It will feel as though there is a breeze in your face. The arrows on the ball indicate the direction the ball is rotating. When the arrows point in the same direction as the ‘wind direction’ a slight vacuum will form.

Nature doesn’t like vacuums and will tend to try and fill it. The way this occurs is by surrounding objects filling the void. In this case, it is the table tennis ball. The ball will tend to drop into the vacuum. This explains why top spun shots will drop quickly.

An underspun ball moving through air:

 

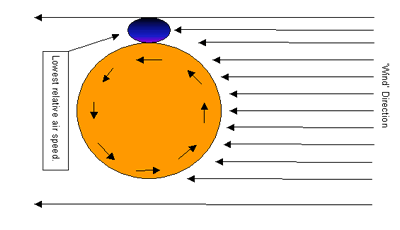With underspin, the vacuum forms at the top of the ball, and ‘sucks’ the ball upwards. The same principle applies with sidespin, except the vacuum forms on the side of the ball, sucking it left or right, depending on the spin put on it.

Also, a slight vacuum forms at the rear of the ball, due to its motion. There is no technique that can overcome this, it’s the nature of anything in motion (ie even a snail sliding across a leaf will have this vacuum). The only thing that can be done is to use a new ball.

Don’t like this explanation? Then try this one on for size.

Why a new ball is preferable to an old one

This is because the additional powdery stuff on the ball causes the air around the ball to be pulled around to the back of it slightly, thereby minimising the vacuum discussed above. The smaller this vacuum, the faster the ball travels. This applies to any projectile. It is for this reason that both table tennis and tennis balls are replaced during tournament play. In tennis, it’s how hairy the ball is that minimises the vacuum.

A new ball is also fractionally heavier than an old one, and when it contacts the table, it will ‘sink’ into the table slightly more than a lighter one. Because of this, the effect of the spin will be more pronounced. The powder also makes the ball ‘grippier’, thereby increasing the effect spin can have on the ball. In Physics terminology, the powder increases the frictional coefficient.

Another interesting fact is that table tennis balls are pressurised slightly. As the balls are used more and more, this pressure leaks out (even if the balls aren’t used, it will occur, but using them speeds up this process). My guess is that with top level play, this drop in pressure would lead to the ball having marginally different playing characteristics.

Reaction Speed in Table Tennis

From a biological perspective, there are limits to how fast the body can react to a stimulus. There is a difference in this time between an audio stimulus and visual stimulus. Technically we respond faster to an audio stimulus than a visual stimulus, 0.18 of a second compared with 0.14 of a second respectively. Therefore, if you can work out EVERYTHING about the shot you need to just by hearing it strike the racquet, you’re 0.04 or four one hundredths of a second faster than anyone else who has ever played table tennis before.

Good players (even average players like myself) can still deduce a lot of what the opposition is doing, simply by listening to the noise the ball makes when it contacts the bat. For instance a brushing noise of the ball on the bat tells you that spin has been put on the ball, hitting a loop will give this effect. A sharper ‘pock’ will tell you that the ball has been struck quite solidly, and will also tell you that they’re using a thin rubber. It is, of course, legal to ask to view the opposition’s bat, so listening to the noise to tell what thickness rubber is being used is just something that can be done.

Some people say that when the ball strikes the table they can tell whether the ball is top spun or under spun. Personally, I can’t, but it wouldn’t surprise me that elite players can.

In Table Tennis, the average total time to react to a shot is usually around 0.25 of a second. With lots of training and lots of practise, this can be reduced to 0.18 of a second. This is one of the big factors in what separates the greats of table tennis, from the top A grade players. In elite levels of the sport, even being the smallest fraction of a second (1/1000ths) starts to make a difference.

T = rF
Torque is a Force that occurs when it is applied at an angle around a fixed point. This is usually a circle. There are several places that I’ve seen Torque used in Table Tennis. Some common places are:

1. Maximising the spin on the ball. By doing this a sphere (the ball) is rotated about a point inside it. This means that the faster the ball is spinning the higher the Torque.
2. Unwinding the body when playing a powerful shot such as a smash. You unwind your hips, then your torso, then your shoulders, upper arm, lower arm and finally wrist. This increases the Radius of the swing. By hitting the ball toward the outer rim of the racquet will also increase the radius. I don’t know if this is used in the game, as doing this would mean the ball is striking the racquet outside of the sweet spot and causing a loss of control.
3. When serving a forehand pendulum serve, one technique is to trick the opponent by minimising the amount of spin put on the ball. This is done by contacting the ball close to the handle, thereby minimising the Radius of the swing.

Technically hitting the ball harder (with a higher velocity) also increases the Torque, as this increase in velocity results in a direct increase in the acceleration of the ball. As F = ma, an increase in a leads to a direct increase in F, which in turn leads to a direct increase in Torque.

i.e.
a = (v – u)/t
F = ma
T = rF

Energy

Energy cannot be observed. Only the results of Energy can be observed. That is, when a ball is hit hard, you are observing the transfer of Energy from the body of the player to the ball to cause that shot, not Energy itself.

Energy is described in two forms (ignoring a smattering of other forms, which, without getting extremely technical in chemistry and nuclear physics, are beyond the scope of this article). These are Potential Energy and Kinetic Energy.

The formulae used are:

Potential Energy:	E = mgh
Kinetic Energy:	E = ½mv2

 

E =	Energy
m =	Mass
g =	The acceleration due to gravity (9.81001 ms-2 to 5 decimal places if you must know)
h =	Height of the object
v =	Velocity

E = mgh

This is a representation of Potential Energy. This represents the ability of the object in question to use Energy. For instance, if a Table Tennis ball was in your hand and you remove your hand quickly, the ball would start to fall (due to gravity). As this occurs, the ball’s potential energy starts to be converted to kinetic energy. When it hits the ground, the kinetic energy starts to change back to potential energy, till the ball reaches the peak of its bounce, and starts to fall again.

Theoretically, this should continue forever, as Energy cannot be created or destroyed (except in a nuclear reaction, which involves what is probably Science’s most famous equation: E = mc²). The reason it doesn’t continue forever is due to air resistance, in the form of friction, and the fact that the collision of the ball and the ground isn’t perfectly elastic (some of the ball’s kinetic energy is converted to heat, when it impacts with the ground, and there is also some friction between the floor and the ball).

If you want to conduct an experiment (you can make quite a bit of money out of this ‘trick’), try dropping a golf ball and a table tennis ball from the same height and see which hits the ground first. Both will strike at the same time, as the resistance due to air is almost exactly equal. Another way is to perform the experiment in a vacuum, though this is a harder to set up. In that case, you can drop a feather and a brick, and the two will strike the ground simultaneously.

This explains why a serve with a high ball toss is more dangerous than one tossed only 6 inches high. The Energy gained by the high toss can be converted to spin or speed when struck by the racquet.

E = ½mv²

This formula shows that the faster you hit the ball, the more Energy the shot will have. If the mass of the bat is high, then it will also result in more Energy in the shot. This is because the mass and energy terms are both directly proportional to the Energy.

Why is the 38mm ball faster than the 40mm ball?

As the 38mm ball has a smaller radius, it also has a lower mass, and therefore a lower Energy due to the equation E = ½mv². This should therefore mean that the overall velocity of the ball is lower. BUT, the 38mm ball is faster than the 40mm ball because the increase in the radius results in an increase in the wind resistance, thus slowing down the 40mm ball. When you deal with objects of low mass like a table tennis ball, air resistance is a major factor in slowing it down.

And that is a basic introduction to the physics of table tennis.

Jonathan Roberts 8)

Copyright 2005 Jonathan Roberts

<Greg’s Note January 2020: Since first publishing this article in 2005, I have received a number of complaints from people claiming that the physics and mathematics contained therein are faulty. My answer to this now is the same as it was then – anyone who thinks this article is wrong is welcome to send me their own version and I’ll put it up on the site for comparison and contrast.>